http://www.autism-society.org/site/PageServer

artikel1

http://www.biomedcentral.com/content/pdf/1744-9081-2-20.pdf

laman web autisme

http://en.wikipedia.org/wiki/Autism

THE IMPACT OF BELIEFS ON THE TEACHING OF MATHEMATICS

THE IMPACT OF BELIEFS ON THE TEACHING OF MATHEMATICS

Paul Ernest

Official reports such as NCTM (1980) Agenda for Action, and the Cockcroft Report (1982) recommend the adoption of a problem solving approach to the teaching of mathematics. Such reforms depend to a large extent on institutional reform: changes in the overall mathematics curriculum. They depend even more essentially on individual teachers changing their approaches to the teaching of mathematics. However the required changes are unlike those of a skilled machine operative, who can be trained to upgrade to a more advanced lathe, for example. A shift to a problem solving approach to teaching requires deeper changes. It depends fundamentally on the teacher's system of beliefs, and in particular, on the teacher's conception of the nature of mathematics and mental models of teaching and learning mathematics. Teaching reforms cannot take place unless teachers' deeply held beliefs about mathematics and its teaching and learning change. Furthermore, these changes in beliefs are associated with increased reflection and autonomy on the part of the mathematics teacher. Thus the practice of teaching mathematics depends on a number of key elements, most notably:

• the teacher's mental contents or schemas, particularly the system of beliefs concerning mathematics and its teaching and learning;
• the social context of the teaching situation, particularly the constraints and opportunities it provides; and
• the teacher's level of thought processes and reflection.

These factors are therefore those which determine the autonomy of the mathematics teacher, and hence also the outcome of teaching innovations - like problem solving - which depend on teacher autonomy for their successful implementation.

The mathematics teacher's mental contents or schemas includes knowledge of mathematics, beliefs concerning mathematics and its teaching and learning, and other factors. Knowledge is important, but it alone is not enough to account for the differences between mathematics teachers. Two teachers can have similar knowledge, but while one teaches mathematics with a problem solving orientation, the other has a more didactic approach. For this reason, the emphasis below is placed on beliefs. The key belief components of the mathematics teacher are the teacher's:

• view or conception of the nature of mathematics,
• model or view of the of the nature of mathematics teaching
• model or view of the process of learning mathematics,

The teacher's conception of the nature of mathematics, is his or her belief system concerning the nature of mathematics as a whole. Such views form the basis of the philosophy of mathematics, although some teacher's views may not have been elaborated into fully articulated philosophies. Teachers' conceptions of the nature of mathematics by no means have to be consciously held views; rather they may be implicitly held philosophies. The importance for teaching of such views of subject matter has been noted both across a range of subjects, and for mathematics in particular (Thom, 1973). Three philosophies are distinguished here because of their observed occurrence in the teaching of mathematics (Thompson 1984), as well as in the philosophy of mathematics and science.

First of all, there is the instrumentalist view that mathematics is an accumulation of facts, rules and skills to be used in the pursuance of some external end. Thus mathematics is a set of unrelated but utilitarian rules and facts.
Secondly, there is the Platonist view of mathematics as a static but unified body of certain knowledge. Mathematics is discovered, not created.
Thirdly, there is the problem solving view of mathematics as a dynamic, continually expanding field of human creation and invention, a cultural product. Mathematics is a process of enquiry and coming to know, not a finished product, for its results remain open to revision.

These three philosophies of mathematics, as psychological systems of belief, can be conjectured to form a hierarchy. Instrumentalism is at the lowest level, involving knowledge of mathematical facts, rules and methods as separate entities. At the next level is the Platonist view of mathematics, involving a global understanding of mathematics as a consistent, connected and objective structure. At the highest level, the problem solving view sees mathematics as a dynamically organised structure located in a social and cultural context.

The model of teaching mathematics is the teacher's conception of the type and range of teaching roles, actions and classroom activities associated with the teaching of mathematics. Many contributing constructs can be specified including unique versus multiple approaches to tasks, and individual versus cooperative teaching approaches. Three different models which can be specified through the teacher's role and intended outcome of instruction are:

TEACHER'S ROLE	INTENDED OUTCOME
1. Instructor:	Skills mastery with correct performance
2. Explainer:	Conceptual understanding with unified knowledge
3. Facilitator:	Confident problem posing and solving

The use of curricular materials in mathematics is also of central, importance in a model of teaching. Three patterns of use are:

1. The strict following of a text or scheme;
2. Modification of the textbook approach, enriched with additional problems and activities;
3. Teacher or school construction of the mathematics curriculum.

Closely associated with the above is the teacher's mental model of the learning of mathematics. This consists of the teacher's view of the process of learning mathematics, what behaviours and mental activities are involved on the part of the learner, and what constitute appropriate and prototypical learning activities. Two of the key constructs for these models are: learning as active construction, as opposed to the passive reception of knowledge; the development of autonomy and child interests in mathematics, versus a view of the learner as submissive and compliant. Using these key constructs the following simplified models can be sketched, based on the child's:

1. compliant behaviour and mastery of skills model,
2. reception of knowledge model,
3. active construction of understanding model,
4. exploration and autonomous pursuit of own interests model.

RELATIONSHIPS BETWEEN BELIEFS, AND THEIR IMPACT ON PRACTICE

The relationships between teachers' views of the nature of mathematics and their models of its teaching and learning are illustrated in the following diagram. It shows how teachers' views

FIG. 1 Relationships between beliefs, and their impact on practice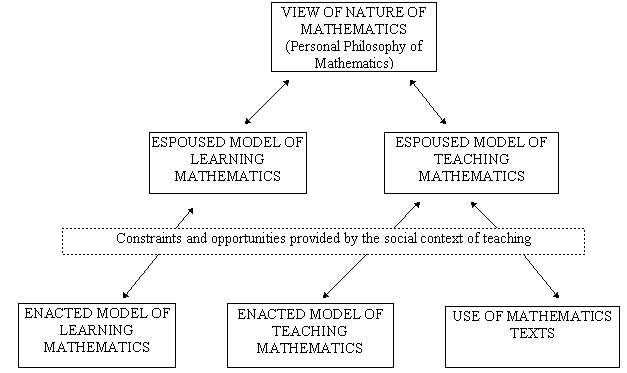

of the nature of mathematics provide a basis for the teachers' mental models of the teaching and learning of mathematics, as indicated by the downward arrows. Thus, for example, the instrumental view of mathematics is likely to be associated with the instructor model of teaching, and with the strict following of a text or scheme. It is also likely to be associated with the child's compliant behaviour and mastery of skills model of learning. Similar links can be made between other views and models, for example:

• Mathematics as a Platonist unified body of knowledge - the teacher as explainer - learning as the reception of knowledge;
• Mathematics as problem solving - the teacher as facilitator - learning as the active construction of understanding, possibly even as autonomous problem posing and solving.

These examples show the links between the teacher's mental models, represented by horizontal arrows in the diagram.

The teacher's mental or espoused models of teaching and learning mathematics, subject to the constraints and contingencies of the school context, are transformed into classroom practices. These are the enacted (as opposed to espoused) model of teaching mathematics, the use of mathematics texts or materials, and the enacted (as opposed to espoused) model of learning mathematics. The espoused-enacted distinction is necessary, because case-studies have shown that there can be a great disparity between a teacher's espoused and enacted models of teaching and learning mathematics (for example Cooney, 1985). Two key causes for the mismatch between beliefs and practices are as follows.

First of all, there is the powerful influence of the social context. This results from the expectations of others including students, parents, peers (fellow teachers) and superiors. It also results from the institutionalised curriculum: the adopted text or curricular scheme, the system of assessment, and the overall national system of schooling. These sources lead the teacher to internalise a powerful set of constraints affecting the enactment of the models of teaching and learning mathematics. The socialisation effect of the context is so powerful that despite having differing beliefs about mathematics and its teaching, teachers in the same school are often observed to adopt similar classroom practices.

Secondly, there is the teacher's level of consciousness of his or her own beliefs, and the extent to which the teacher reflects on his or her practice of teaching mathematics. Some of the key elements in the teacher's thinking - and its relationship to practice - are the following.

• Awareness of having adopted specific views and assumptions as to the nature of mathematics and its teaching and learning.
• The ability to justify these views and assumptions.
• Awareness of the existence of viable alternatives.
• Context-sensitivity in choosing and implementing situationally appropriate teaching and learning strategies in accordance with his or her own views and models.
• Reflexivity: being concerned to reconcile and integrate classroom practices with beliefs; and to reconcile conflicting beliefs themselves.

These elements of teacher's thinking are likely to be associated with some of the beliefs outlined above, at least in part. Thus, for example, the adoption of the role of facilitator in a problem-solving classroom requires reflection on the roles of the teacher and learner, on the context suitability of the model, and probably also on the match between beliefs and practices. The instrumental view and the associated models of teaching and learning, on the other hand, requires little self consciousness and reflection, or awareness of the existence of viable alternatives.

I have argued that mathematics teachers' beliefs have a powerful impact on the practice of teaching. During their transformation into practice, two factors affect these beliefs: the constraints and opportunities of the social context of teaching, and the level of the teacher's thought. Higher level thought enables the teacher to reflect on the gap between beliefs and practice, and to narrow it. The autonomy of the mathematics teacher depends on all three factors: beliefs, social context, and level of thought. For beliefs can determine, for example, whether a mathematics text is used uncritically or not, one of the key indicators of autonomy. The social context clearly constrains the teacher's freedom of choice and action, restricting the ambit of the teacher's autonomy. Higher level thought, such as self-evaluation with regard to putting beliefs into practice, is a key element of autonomy in teaching. Only by considering all three factors can we begin to do justice to the complex notion of the autonomous mathematics teacher.

REFERENCES

Cockcroft, W. H. (1982) Mathematics Counts, HMSO, London.
Cooney, T. J. (1985) Journal for Research in Mathematics Education, 16(5), 324-336.
NCTM (1980) Agenda for Action, NCTM, Reston, Virginia.
Thom, R. (1973) in Howson, A. G. (ed.) Developments in Mathematical Education, Cambridge, 194-209.
Thompson, A. G. (1984) Educational Studies in Mathematics, 15, 105-127.

---

This paper was presented as ‘The Impact of Beliefs on the Teaching of Mathematics’ at 6th International Congress of Mathematical Education, Budapest, August 1988. Printed as ‘The Impact of Beliefs on the Teaching of Mathematics’, in P. Ernest, Ed. Mathematics Teaching: The State of the Art, London, Falmer Press, 1989: 249-254. Printed slightly abridged as ‘The impact of beliefs on teaching’, in C. Keitel with P. Damerow, A. Bishop, and P. Gerdes, Eds. Mathematics Education and Society, Paris, UNESCO, 1989: 99-101. Reprinted as ‘The Impact of Beliefs on the Teaching of Mathematics’, in Bloomfield, A. and Harries, T. Eds (1994) Teaching and Learning Mathematics, Derby: Association of Teachers of Mathematics.